To simplify a fraction, divide the top and bottom by the highest number that
can divide into both numbers exactly.Simplifying Fractions
Simplifying (or reducing) fractions means to make the fraction as simple as possible. Why say four-eighths (4/8) when you really mean half (1/2) ?
4/8 (Four-Eights)

2/4(Two-Quarters)

1/2(One-Half)

How do I Simplify a Fraction ?
There are two ways to simplify a fraction:
Method 1
Try dividing both the top and bottom of the fraction until you can't go any further (try dividing by 2,3,5,7,... etc).
Method 2
Divide both the top and bottom of the fraction by the Greatest Common Factor, (you have to work it out first!).
Fractions may have numerators and denominators that are composite numbers (numbers that has more factors than 1 and itself).
How to simplify a fraction:
1.Find a common factor of the numerator and denominator. A common factor is a number that will divide into both numbers evenly. Two is a common factor of 4 and 14.
Divide both the numerator and denominator by the common factor.
Repeat this process until there are no more common factors.The fraction is simplified when no more common factors exist.
Another method to simplify a fraction
2.Find the Greatest Common Factor (GCF) of the numerator and denominator
Divide the numerator and the denominator by the GCF.
Operations in fractions
Multiplying Fractions
Multiplying two fractions is the easiest of any of the operations.
Rule for Multiplication of Fractions
When multiplying fractions, you simply multiply the numerators together and then multiply the denominators together. Simplify the result.

This works whether the denominators are the same or not.
So, if you wish to multiply the fractions 3/2 and 4/3 together you get 12/6.
As with any solution, you should report the answer in simplified form. The fraction 12/6 can be simplified to 2.

You should recall that any number divided by itself is 1, so 6/6=1. In other words, if you find the same number on both the top and the bottom of a fraction, you can cancel it out.
Example
What do you get when you multiply 1/2 and 3/7?
The result of multiplying these two fractions is 3/14.

The fraction 3/14 cannot be simplified any further; it is in its simplest form.
Dividing Fractions
Dividing one fraction by another is almost as easy as multiplying two fractions. It even involves multiplying fractions! First, let's look at how division of two fractions may be represented. If we wish to divide 3/5 by 2/3, we could write that as:
 or
or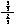
Rule for Division of Fractions
When you divide two fractions, you take the reciprocal of the second fraction, or bottom fraction, and multiply. (Taking the reciprocal of a fraction means to flip it over.)

As with multiplication, this works whether the denominators are the same or not.
So, if you wish to divide the fraction 3/2 by 4/3, you get the result shown at the right. As with any solution, you should report the answer in its simplified form. In this case, 9/8 is in its simplest form.

Example
What do you get when you divide 12/17 by 6/7?
The answer is 14/17.
We take the reciprocal of the second fraction and multiplying it by the first. We get 82/102, which, however, is not in its simplified form.

One easy way to simplify this fraction is go back to the step before the numerator and denominator were multiplied.

To reduce a fraction to its simplest form, we need to find the prime factors of both the numerator and denominator (This was shown in the unit on Equivalent Fractions). When we do this for the numerator and denominator we find we can cancel out a 2 and a 3 from the top and bottom. This gives us the result 14/17.

Adding and Subtracting Fractions
When adding and subtracting fractions, the fractions being added or subtracted must have the same denominator. When denominators are different, you will need to convert each fraction into an equivalent fraction by finding the least common denominator (LCD) for the fractions. The two new fractions should have the same denominator, making them easy to add or subtract. (Determining the LCD of a set of fractions was reviewed in the unit Comparing Fractions.)
Rule for Addition of Fractions
When adding fractions, you must make sure that the fractions being added have the same denominator. If they do not, find the LCD for the fractions and put each in its equivalent form. Then, simply add the numerators of the fractions.

Rule for Subtraction of Fractions
When subtracting fractions, you must make sure that the fractions being subtracted have the same denominator. If they do not, find the LCD for the fractions and put each in its equivalent form. Then, simply subtract the numerators of the fractions.

This rule can be broken down into several steps:
1. Determine whether the fractions have the same denominator.
If the denominators are the same, move to step 4.2. If the denominators are different, find the LCD for the fractions being added.
3. Find the equivalent fractions with the LCD in the denominator.
4. Add or subtract the numerators of the fractions.
5. Simplify the resulting fraction.
1. If we have the fractions 1/6 and 2/6, and wish to add them, we follow our steps:
4. Determine whether the fractions have the same denominator. If the denominators are the same, move to step 4.
 5. Simplify the resulting fraction. This fraction can be simplified to 1/2.
5. Simplify the resulting fraction. This fraction can be simplified to 1/2.The fractions 1/6 and 2/6 have the same denominator, so we can move to step 4.

Visually, this would look like:

Now let's try an example.
Example of Adding Fractions
What is the sum of 3/4 and 1/3?
The answer is 13/12.
Following the steps:
1. Determine whether the fractions have the same denominator.
If the denominators are the same, move to step 4.
First, you should notice that the two fractions do not have the same denominator. This means we need to find the LCD for the two fractions.
2. Find the LCD for the fractions being added.
a. Write the prime factors for the denominator of each fraction.
The prime factors of 4 are: 2 and 2.
The prime factor of 3 is: 3
2. Note all prime factors that occur. For each prime factor that occurs, determine in which denominator it occurs the most. Write down the prime factor the number of times it occurs in that one denominator.
The prime factors that occur are 2, 2, and 3.
3. Calculate the LCD of your fractions. To do this, multiply the factors selected in step 2b.
2 x 2 x 3= 12,
12 is our LCD.
4. Find the equivalent fractions that have the LCD in the denominator.
Let's start with 3/4. The prime factor missing from this denominator is a 3. So, 3 is the multiplier for 3/4.

For the fraction 1/3, the prime factors that are missing are 2 and 2. Since 2 x 2= 4, 4 is the multiplier for the fraction 1/3.

Add the numerators of the fractions.
Now that we have found the fractions that are equivalent to the ones we are adding, and these have the same denominator, we can add the fractions together.
We can see that the fraction we are adding are 9/12 and 4/12, which equals 13/12.
5. Simplify the resulting fraction.
The answer of 13/12 is in its simplest form.
The steps for subtracting fractions are the same as for addition. The only difference is substituting subtraction for addition. If we wish to subtract 1/8 from 4/8, we can follow the steps outlined above.
1. Determine whether the fractions have the same denominator. If the denominators are the same, move to step 4.
They are the same, so we can skip to step 4.
4. Subtract the numerators of the fractions.

5. Simplify the resulting fraction.
This fraction, 3/8, is in its simplest form.
As you can see, addition and subtraction of fractions is similar to adding and subtracting whole numbers. The important point is to be sure the fractions being added or subtracted have the same denominator.
Reference:
http://www.mathsisfun.com/simplifying-fractions.html
http://www.aaamath.com/fra66h-simpfrac.html
http://cstl.syr.edu/FIPSE/fracunit/Opfrac/Opfrac.htm
No comments:
Post a Comment